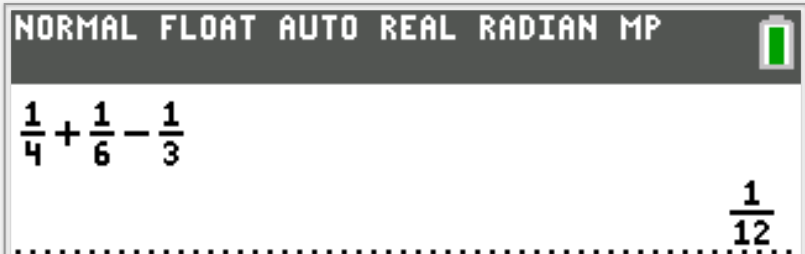
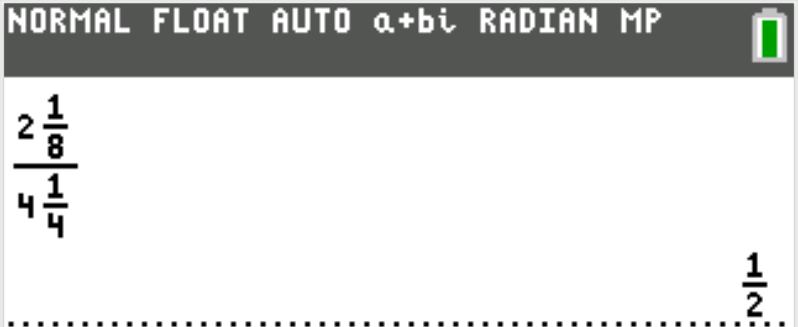
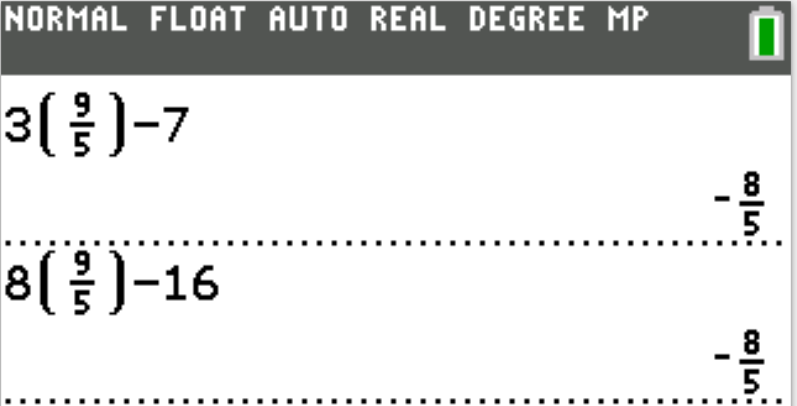
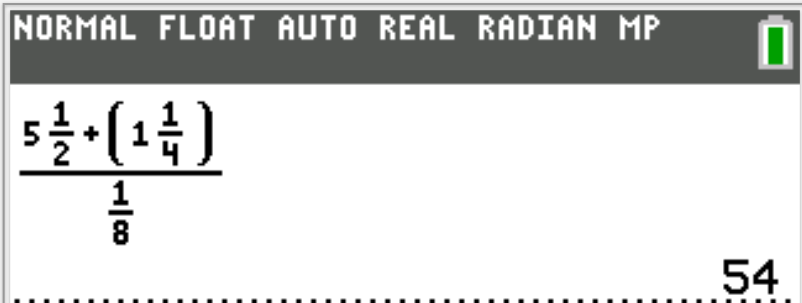
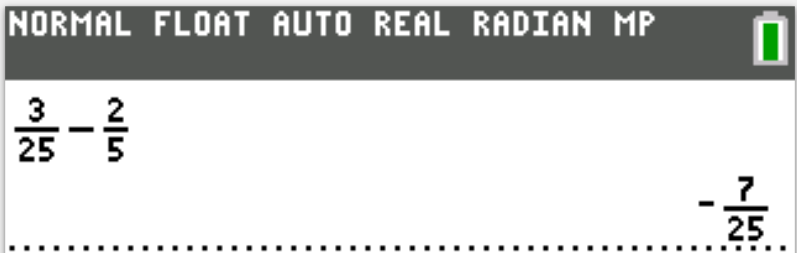
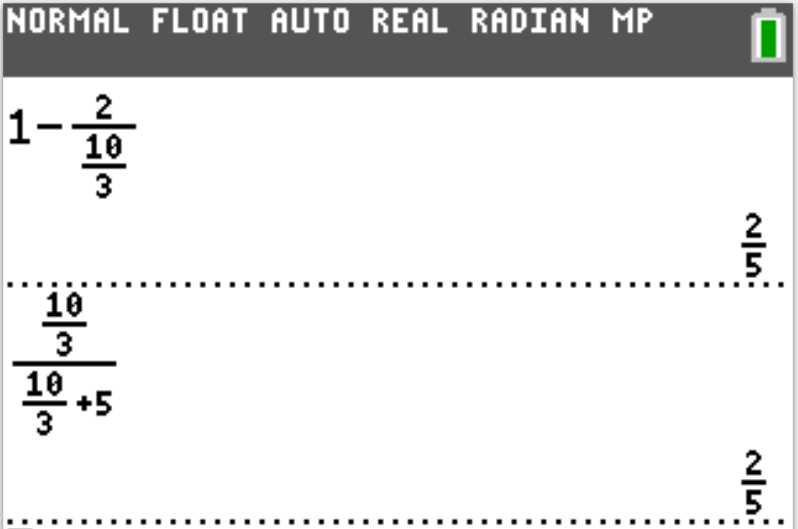
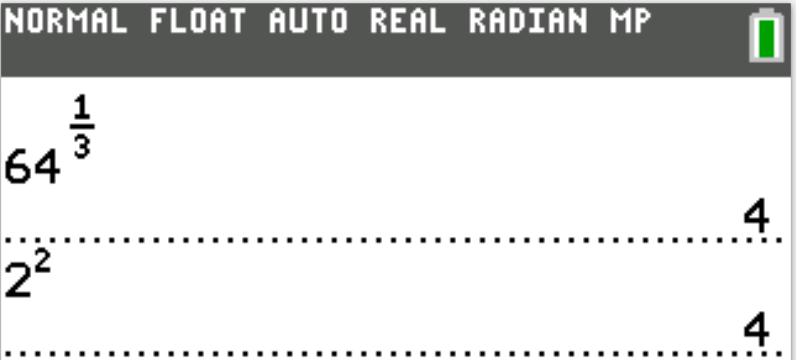
Expressions and Equations
Welcome to Our Site
I greet you this day,
For the Classic ACT exam:
The ACT Mathematics test is a timed exam...60 questions in 60 minutes
This implies that you have to solve each question in one minute.
Each of the first 20 questions (less challenging) will typically take less than a minute a solve.
Each of the next 20 questions (medium challenging) may take about a minute to solve.
Each of the last 20 questions (more challenging) may take more than a minute to solve.
The goal is to maximize your time.
You use the time saved on the questions you solve in less than a minute to solve questions that will take more
than a minute.
So, you should try to solve each question correctly and timely.
So, it is not just solving a question correctly, but solving it correctly on time.
Please ensure you attempt all ACT questions.
There is no negative penalty for a wrong answer.
Also: please note that unless specified otherwise, geometric figures are drawn to scale. So, you can figure out
the correct answer by eliminating the incorrect options.
Other suggestions are listed in the solutions/explanations as applicable.
These are the solutions to the ACT past questions on the topics: Expressions and Equations.
When applicable, the TI-84 Plus CE calculator (also applicable to TI-84 Plus calculator) solutions are provided
for some questions.
The link to the video solutions will be provided for you. Please
subscribe to the YouTube channel to be notified of upcoming livestreams. You are welcome to ask questions during
the video livestreams.
If you find these resources valuable and helpful in your passing the
Mathematics test of the ACT, please consider making a donation:
Cash App: $ExamsSuccess or
cash.app/ExamsSuccess
PayPal: @ExamsSuccess or
PayPal.me/ExamsSuccess
Google charges me for the hosting of this website and my other
educational websites. It does not host any of the websites for free.
Besides, I spend a lot of time to type the questions and the solutions well.
As you probably know, I provide clear explanations on the solutions.
Your donation is appreciated.
Comments, ideas, areas of improvement, questions, and constructive
criticisms are welcome.
Feel free to contact me. Please be positive in your message.
I wish you the best.
Thank you.
English to Math: Something About Word Problems
(1.) Word problems are written in standard British English.
(2.) Some word problems are very lengthy and some are unnecessary.
Those ones are meant to discourage you from even trying.
(3.) Word problems in Mathematics demonstrate the real-world applications of mathematical concepts.
(4.) Embrace word problems. See it as writing from English to Math.
Take time to:
(a.) Read to understand. Paraphrase and shorten long sentences as necessary.
(b.) Re-read and note/underline the vocabulary "math" terms written in English.
(c.) Translate/Write "important" sentences one at a time.
(d.) Review what you wrote to ensure correctness.
(e.) Solve the math, and check your solution in the word problem.
Does that solution makes sense? If it does, you are probably correct. If it does not, please re-do it.
| English | Math |
|---|---|
| $7$ more than a number, $p$ | $7 + p$ |
| $7$ increased by a number, $q$ | $7 + q$ |
| $7$ added to a number, $r$ | $7 + r$ |
| A number, $g$ added to $7$ | $g + 7$ |
| The sum of $7$ and a number $t$ | $7 + t$ |
| $7$ less than a number, $p$ | $p - 7$ |
| $7$ decreased by a number, $q$ | $7 - q$ |
| A number, $r$ decreased by $7$ | $r - 7$ |
| $7$ subtracted from a number, $e$ | $e - 7$ |
| A number, $t$ subtracted from $7$ | $7 - t$ |
| The difference of $7$ and a number, $u$ | $7 - u$ |
| The difference of a number, $v$ and $7$ | $v - 7$ |
| $7$ times a number, $p$ | $7 * p$ = $7p$ |
| $7$ multiplied by a number, $q$ | $7 * q$ = $7q$ |
| A number, $r$ multiplied by $7$ | $r * 7$ = $7r$ |
| The product of a $7$ and a number, $h$ | $7 * h$ = $7h$ |
| The product of a number $t$ and $7$ | $t * 7$ = $7t$ |
| $7$ divided by a number, $p$ | $7 \div p$ |
| A number, $q$ divided by $7$ | $q \div 7$ |
| $7$ divides a number, $r$ | $r \div 7$ |
| A number, $m$ divides $7$ | $7 \div m$ |
| The quotient of $7$ and a number, $t$ | $7 \div t$ |
| The quotient of a number, $u$ and $7$ | $u \div 7$ |
| A number, $d$ decreased by the sum of the number and five | $d - (d + 5)$ |
| Three times the product of negative two and a number, $c$ | $3(-2c)$ |
| "is" or "equal to" or "equals" | $=$ |
| $3$ consecutive integers beginning with $3$ | $3, 4, 5$ |
| $3$ consecutive integers beginning with $-3$ | $-3, -2, -1$ |
| $3$ consecutive integers beginning with $p$ | $p, (p + 1), (p + 2)$ |
| $3$ consecutive even integers beginning with $8$ | $8, 10, 12$ |
| $3$ consecutive even integers beginning with $-8$ | $-8, -6, -4$ |
| $3$ consecutive even integers beginning with $q$ | $q, (q + 2), (q + 4)$ |
| $3$ consecutive odd integers beginning with $7$ | $7, 9, 11$ |
| $3$ consecutive odd integers beginning with $-7$ | $-7, -5, -3$ |
| $3$ consecutive odd integers beginning with $r$ | $r, (r + 2), (r + 4)$ |
| The smaller of three consecutive integers beginning with $p$ | $p$ |
| The middle of three consecutive integers beginning with $p$ | $p + 1$ |
| The larger of three consecutive integers beginning with $p$ | $p + 2$ |
| The median of five consecutive odd integers beginning with $p$ | $p + 4$ |
| The larger of five consecutive even integers beginning with $p$ | $p + 8$ |
| The sum of three consecutive integers is $12$ |
Let the first integer = $p$ $p + (p + 1) + (p + 2) = 12$ |
| The sum of three consecutive even integers is $12$ |
Let the first even integer = $p$ $p + (p + 2) + (p + 4) = 12$ |
| The sum of three consecutive odd integers is $12$ |
Let the first odd integer = $k$ $k + (k + 2) + (k + 4) = 12$ |
| Twice a number, $p$ | $2 * p$ = $2p$ |
| Double a number, $d$ | $d * 2$ = $2d$ |
| Thrice a number, $r$ | $3 * r$ = $3r$ |
| Triple a number, $c$ | $c * 3$ = $3c$ |
| Quadruple a number, $p$ | $4 * p$ = $4p$ |
| Multiplicative inverse or Reciprocal of a number, $p$ | $\dfrac{1}{p}$ |
| $5$ less than twice a number, $c$ |
Twice a number, $c$ = $2c$ $5$ less than $2c$ = $2c -5$ $2c - 5$ |
| $4$ more than twice as many tickets, $t$ |
Twice as many tickets, $t$ = $2t$ $4$ more than $2t$ = $4 + 2t$ $4 + 2t$ |
| The difference between the product of seven and a number, $k$; and twice the number. |
Product of seven and $k$ = $7k$ Twice $k$ = $2 * k = 2k$ Difference between $7k$ and $2k$ = $7k - 2k$ $7k - 2k$ |
| The product of seven less than the temperature, $t$ and three |
Seven less than $t$ = $t - 7$ Product of $t - 7$ and three = $3(t - 7)$ $3(t - 7)$ |
| The difference between the profit, $P$ divided by four and nine |
$P$ divided by four = $\dfrac{P}{4}$ Difference between $\dfrac{P}{4}$ and nine = $\dfrac{P}{4} - 9$ $\dfrac{P}{4} - 9$ |
| The difference between twelve times a number, $d$; and five more than three times the number. |
Twelve times $d$ = $12d$ Three times $d$ = $3 * d = 3d$ Five more than $3d$ = $5 + 3d$ Difference between $12d$ and $5 + 3d$ = $12d - (5 + 3d)$ $12d - (5 + 3d)$ |
| A number, $p$ decreased by the difference between ten and the number. |
Difference between ten and $p$ = $10 - p$ $p$ decreased by $10 - p$ = $p - (10 - p)$ $p - (10 - p)$ |
| The difference between the square of a number, $m$ and six times the number. |
Square of $m$ = $m^2$ Six times $m$ = $6 * m = 6m$ Difference between $m^2$ and $6m$ = $m^2 - 6m$ $m^2 - 6m$ |
| The cube of the sum of a number, $p$ and seven. |
Sum of $p$ and seven = $p + 7$ Cube of $p + 7$ = $(p + 7)^3$ $(p + 7)^3$ |
| The sum of a number, $k$ increased by nine and a number, $d$ decreased by seven. |
$k$ increased by nine = $k + 9$ $d$ decreased by seven = $d - 7$ Sum of $k + 9$ and $d - 7$ = $(k + 9) + (d - 7)$ $(k + 9) + (d - 7)$ |
| Twelve less than the quotient of the square of a number, $e$ and eight. |
Square of $e$ = $e^2$ Quotient of $e^2$ and eight = $\dfrac{e^2}{8}$ Twelve less than $\dfrac{e^2}{8}$ = $\dfrac{e^2}{8} - 12$ $\dfrac{e^2}{8} - 12$ |
| Complement of an angle, $\theta$ | $90 - \theta$ |
| Suppleement of an angle, $\theta$ | $180 - \theta$ |
Factoring Formulas
$ \underline{Difference\;\;of\;\;Two\;\;Squares} \\[3ex] (1.)\;\;x^2 - y^2 = (x + y)(x - y) \\[5ex] \underline{Difference\;\;of\;\;Two\;\;Cubes} \\[3ex] (2.)\;\; x^3 - y^3 = (x - y)(x^2 + xy + y^2) \\[5ex] \underline{Sum\;\;of\;\;Two\;\;Cubes} \\[3ex] (3.)\;\; x^3 + y^3 = (x + y)(x^2 - xy + y^2) \\[4ex] $Formulas Relating to Quadratic Expressions and Equations
$ (1.)\;\; Discriminant = b^2 - 4ac \\[5ex] (2.)\;\; \text{Quadratic Formula}:\;\; x = \dfrac{-b \pm \sqrt{b^2 - 4ac}}{2a} \\[6ex] (3.)\;\; \text{Sum of roots} = -\dfrac{b}{a} \\[5ex] (4.)\;\; \text{Product of roots} = \dfrac{c}{a} $$ A.\;\; \dfrac{16}{3} \\[5ex] B.\;\; \dfrac{32}{3} \\[5ex] C.\;\; \dfrac{37}{3} \\[5ex] D.\;\; \dfrac{38}{3} \\[5ex] E.\;\; \dfrac{52}{3} \\[5ex] $
$ \dfrac{3x - 6}{2} + 5 = 18 \\[5ex] \dfrac{3x - 6}{2} = 18 - 5 \\[5ex] \dfrac{3x - 6}{2} = 13 \\[5ex] 3x - 6 = 2(13) \\[3ex] 3x - 6 = 26 \\[3ex] 3x = 26 + 6 \\[3ex] 3x = 32 \\[3ex] x = \dfrac{32}{3} \\[5ex] $ Check
| LHS | RHS |
|---|---|
| $ \dfrac{3x - 6}{2} + 5 \\[5ex] \dfrac{3\left(\dfrac{32}{3}\right) - 6}{2} + 5 \\[8ex] \dfrac{32 - 6}{2} - 5 \\[5ex] \dfrac{26}{2} - 5 \\[5ex] 13 - 5 \\[3ex] 8 $ | 18 |
$ F.\;\; \dfrac{1}{12} \\[5ex] G.\;\; \dfrac{1}{9} \\[5ex] H.\;\; \dfrac{1}{7} \\[5ex] J.\;\; \dfrac{1}{5} \\[5ex] K.\;\; \dfrac{3}{4} \\[5ex] $
Let that number be p
$ \dfrac{1}{3} + p = \dfrac{1}{4} + \dfrac{1}{6} \\[5ex] p = \dfrac{1}{4} + \dfrac{1}{6} - \dfrac{1}{3} \\[5ex] \text{LCD of 4, 6, 3} = 12 \\[3ex] \implies \\[3ex] p = \dfrac{3 + 2 - 4}{12} \\[5ex] p = \dfrac{1}{12} $
$ A.\;\; \dfrac{1}{4} \\[5ex] B.\;\; \dfrac{1}{2} \\[5ex] C.\;\; 2 \\[3ex] D.\;\; 2\dfrac{1}{8} \\[5ex] E.\;\; 2\dfrac{1}{2} \\[5ex] $
Let the fraction be p
$ p \;\;of\;\; 4\dfrac{1}{4} \;\;is\;\; 2\dfrac{1}{8} \\[5ex] p \cdot \dfrac{17}{4} = \dfrac{17}{8} \\[5ex] p = \dfrac{17}{8} \div \dfrac{17}{4} \\[5ex] p = \dfrac{17}{8} \cdot \dfrac{4}{17} \\[5ex] p = \dfrac{1}{2} $
$ A.\;\; \dfrac{7}{32} \\[5ex] B.\;\; \dfrac{1}{8} \\[5ex] C.\;\; -\dfrac{1}{8} \\[5ex] D.\;\; -\dfrac{5}{4} \\[5ex] E.\;\; -\dfrac{29}{32} \\[5ex] $
$ y - x \\[3ex] = -\dfrac{11}{16} - \dfrac{18}{32} \\[5ex] = -\dfrac{22}{32} - \dfrac{18}{32} \\[5ex] = \dfrac{-22 - 18}{32} \\[5ex] = -\dfrac{40}{32} \\[5ex] = -\dfrac{40 \div 8}{32 \div 8} \\[5ex] = -\dfrac{5}{4} $
$ F.\;\; -\dfrac{23}{5} \\[5ex] G.\;\; -\dfrac{23}{11} \\[5ex] H.\;\; -\dfrac{9}{5} \\[5ex] J.\;\; \dfrac{9}{5} \\[5ex] K.\;\; \dfrac{23}{11} \\[5ex] $
$ 3x - 7 = 8x - 16 \\[3ex] 8x - 16 = 3x - 7 \\[3ex] 8x - 3x = -7 + 16 \\[3ex] 5x = 9 \\[3ex] x = \dfrac{9}{5} \\[5ex] $ Check
| LHS | RHS |
|---|---|
| $ 3x - 7 \\[3ex] 3\left(\dfrac{9}{5}\right) - 7 \\[5ex] \dfrac{27}{5} - \dfrac{35}{5} \\[5ex] \dfrac{27 - 35}{5} \\[5ex] -\dfrac{8}{5} $ | $ 8x - 16 \\[3ex] 8\left(\dfrac{9}{5}\right) - 16 \\[5ex] \dfrac{72}{5} - \dfrac{80}{5} \\[5ex] \dfrac{72 - 80}{5} \\[5ex] -\dfrac{8}{5} $ |
$ F.\;\; -\dfrac{41}{20} \\[5ex] G.\;\; -\dfrac{41}{52} \\[5ex] H.\;\; -\dfrac{5}{16} \\[5ex] J.\;\; \dfrac{31}{52} \\[5ex] K.\;\; \dfrac{41}{20} \\[5ex] $
$ x = -4 \\[3ex] \dfrac{-5 + 9x}{x^2 - 9x} \\[6ex] \dfrac{-5 + 9(-4)}{(-4)^2 - 9(-4)} \\[6ex] \dfrac{-5 - 36}{16 + 36} \\[5ex] -\dfrac{41}{52} $
Each camper will get a small bag filled with $\dfrac{1}{8}$ pound of the trail mix.
What is the maximum number of bags of trail mix that the camp counselors can fill?
$ A.\;\; 10 \\[3ex] B.\;\; 34 \\[3ex] C.\;\; 44 \\[3ex] D.\;\; 50 \\[3ex] E.\;\; 54 \\[3ex] $
Combine $5\dfrac{1}{2}$ pounds of peanuts with $1\dfrac{1}{4}$ pounds of cashews
= $5\dfrac{1}{2}\;lb + 1\dfrac{1}{4}\;lb$
Let the maximum number of bags of trail mix that the camp counselors can fill = p
Each camper will get a small bag filled with $\dfrac{1}{8}$ pound of the trail mix = $\dfrac{1}{8}\;lb \cdot p$
This implies that:
$ \dfrac{1}{8}p = 5\dfrac{1}{2} + 1\dfrac{1}{4} \\[5ex] \dfrac{p}{8} = \dfrac{11}{2} + \dfrac{5}{4} \\[5ex] \dfrac{p}{8} = \dfrac{22}{4} + \dfrac{5}{4} \\[5ex] \dfrac{p}{8} = \dfrac{22 + 5}{4} \\[5ex] \dfrac{p}{8} = \dfrac{27}{4} \\[5ex] p = \dfrac{8 \cdot 27}{4} \\[5ex] p = 54 $
$ F.\;\; \dfrac{10}{3} \\[5ex] G.\;\; \dfrac{13}{25} \\[5ex] H.\;\; \dfrac{3}{10} \\[5ex] J.\;\; \dfrac{1}{20} \\[5ex] K.\;\; -\dfrac{7}{25} \\[5ex] $
$ x + \dfrac{2}{5} = \dfrac{3}{25} \\[5ex] x = \dfrac{3}{25} - \dfrac{2}{5} \\[5ex] = \dfrac{3}{25} - \dfrac{10}{25} \\[5ex] = \dfrac{3 - 10}{25} \\[5ex] = -\dfrac{7}{25} $
The paper towels come in boxes of 6 rolls with a shipping weight of 6.8 pounds.
The cost for a box of 6 rolls of this paper towel is $15.99 plus the shipping cost, given in the table below.
| Number of boxes shipped | Shipping cost per box |
|---|---|
|
1 – 10 11 – 99 100 or more |
$13.25 $12.00 $10.00 |
The diameter of each cylindrical roll is 6 inches.
Each roll has a cylindrical hole 1.5 inches in diameter, and each roll has a height of 11 inches, as shown in the diagrams below.
The total weight of the box and packaging material is 1 pound.
Which of the following numeric expressions represents the weight, in pounds, of 1 roll of these paper towels?
$ F.\;\; \dfrac{6.8 - 1}{6} \\[5ex] G.\;\; \dfrac{6.8}{6} \\[5ex] H.\;\; \dfrac{6.8 + 1}{6} \\[5ex] J.\;\; 6(6.8) \\[3ex] K.\;\; 6(6.8 - 1) \\[3ex] $
The total weight of the box and packaging material is 1 pound.
The paper towels come in boxes of 6 rolls with a shipping weight of 6.8 pounds.
Let the weight of 1 roll of the 6 rolls of paper towels = w
This implies that the weight of 6 rolls of paper towels = 6w
The weight of the 6 rolls of paper towels + the total weight of the box and packaging material = shipping weight
$ 6w + 1 = 6.8 \\[3ex] 6w = 6.8 - 1 \\[3ex] w = \dfrac{6.8 - 1}{6} $
$ A.\;\; -\dfrac{10}{7} \\[5ex] B.\;\; -1 \\[3ex] C.\;\; 3 \\[3ex] D.\;\; \dfrac{10}{3} \\[5ex] E.\;\; 4 \\[3ex] $
$ 1 - \dfrac{2}{x} = \dfrac{x}{x + 5} \\[5ex] LCD = x(x + 5) \\[3ex] x(x + 5) \cdot 1 - x(x + 5) \cdot \dfrac{2}{x} = x(x + 5) \cdot \dfrac{x}{x + 5} \\[5ex] x^2 + 5x - 2(x + 5) = x^2 \\[3ex] x^2 + 5x - 2x - 10 - x^2 = 0 \\[3ex] 3x = 10 \\[3ex] x = \dfrac{10}{3} $
$LHS = RHS = \dfrac{2}{5}$
A. {−1, 0, 1}
B. {−1, 0}
C. {0, 1}
D. {−1}
E. {0}
$ c^2 + c^9 = 0 \\[3ex] c^2(1 + c^7) = 0 ...\text{Factored by GCF} \\[3ex] c^2 = 0 \hspace{1em}OR\hspace{1em} 1 + c^7 = 0 \\[3ex] c = \sqrt{0} \hspace{1em}OR\hspace{1em} c^7 = -1 \\[3ex] c = 0 \hspace{1em}OR\hspace{1em} c = \sqrt[7]{-1} \\[3ex] c = 0 \hspace{1em}OR\hspace{1em} c = -1 \\[3ex] c = \{-1, 0\} $
$ F.\;\; \dfrac{1}{3} \\[5ex] G.\;\; 2 \\[3ex] H.\;\; 4 \\[3ex] J.\;\; 6 \\[3ex] K.\;\; 8 \\[3ex] $
$ 64^{\dfrac{1}{3}} = 2^x \\[5ex] 2^x = 64^{\dfrac{1}{3}} \\[5ex] 2^x = \sqrt[3]{64} ...Law\;7...Exp \\[3ex] 2^x = 4 \\[3ex] 2^x = 2^2 \\[3ex] \text{same base; equate exponents} \\[3ex] x = 2 $
$ A.\;\; -2 \\[3ex] B.\;\; -0.5 \\[3ex] C.\;\; 0.5 \\[3ex] D.\;\; 1.1 \\[3ex] E.\;\; 2 \\[3ex] $
$ 9 - 11x = 13 - 9x \\[3ex] 9 - 13 = -9x + 11x \\[3ex] -4 = 2x \\[3ex] 2x = -4 \\[3ex] x = -\dfrac{4}{2} \\[5ex] x = -2 $
$ F.\;\; 1 \\[3ex] G.\;\; 3 \\[3ex] H.\;\; \dfrac{1}{2} \\[5ex] J.\;\; \dfrac{3}{2} \\[5ex] K.\;\; \dfrac{5}{2} \\[5ex] $
$ 2x^2 + 5x = 3 \\[3ex] 2x^2 + 5x - 3 = 0 \\[3ex] 2x^2(-3) = -6x^2 \\[3ex] \text{Factors are: } 6x \;\;and\;\; -x \\[3ex] 2x^2 + 6x - x - 3 = 0 \\[3ex] 2x(x + 3) - 1(x + 3) = 0 \\[3ex] (x + 3)(2x - 1) = 0 \\[3ex] x + 3 = 0 \hspace{2em}OR\hspace{2em} 2x - 1 = 0 \\[3ex] x = -3 \hspace{2em}OR\hspace{2em} 2x = 1 \\[3ex] x = -3 \hspace{2em}OR\hspace{2em} x = \dfrac{1}{2} \\[5ex] But\;\; x \gt 0 ...Given \\[3ex] \therefore x = \dfrac{1}{2} $
$ F.\;\; \dfrac{1}{2} \\[3ex] G.\;\; 1\\[3ex] H.\;\; 2 \\[3ex] J.\;\; 4 \\[3ex] K.\;\; 16 \\[3ex] $
$ \left(\sqrt{8a}\right)\left(\sqrt{2a}\right) = 16 \\[3ex] \sqrt{8 \cdot 2 \cdot a \cdot a} = 16 \\[3ex] \sqrt{16 \cdot a^2} = 16 \\[3ex] 4a = 16 \\[3ex] a = \dfrac{16}{4} \\[5ex] a = 4 $
