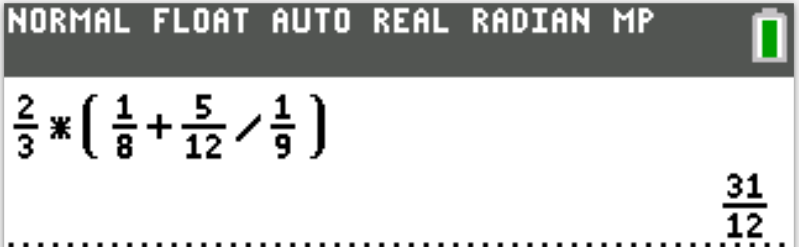
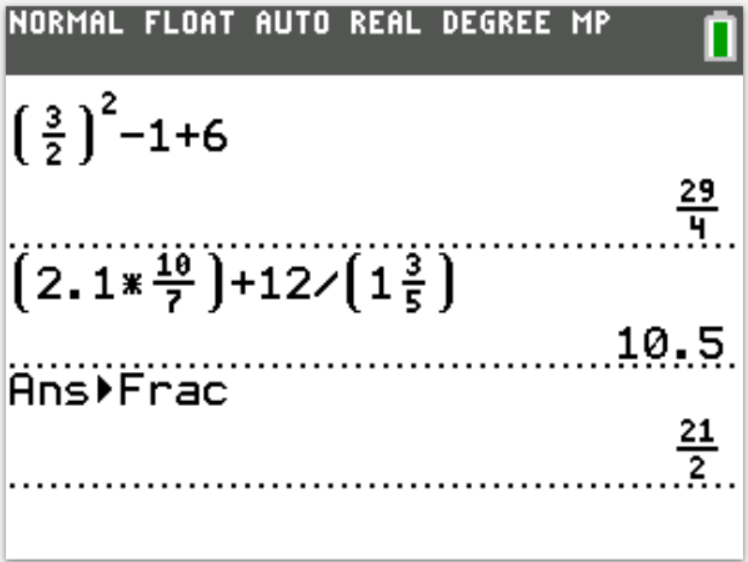
Numbers, Fractions, Decimals, and Percents
Welcome to Our Site
I greet you this day,
These are the solutions to the CSEC past questions on Numbers, Fractions, Decimals, and Percents.
The TI-84 Plus CE shall be used for applicable questions.
The link to the video solutions will be provided for you. Please subscribe to the YouTube channel to be notified
of upcoming livestreams.
You are welcome to ask questions during the video livestreams.
If you find these resources valuable and if any of these resources were helpful in your passing the
Mathematics exams of CSEC, please consider making a donation:
Cash App: $ExamsSuccess or
cash.app/ExamsSuccess
PayPal: @ExamsSuccess or
PayPal.me/ExamsSuccess
Google charges me for the hosting of this website and my other
educational websites. It does not host any of the websites for free.
Besides, I spend a lot of time to type the questions and the solutions well.
As you probably know, I provide clear explanations on the solutions.
Your donation is appreciated.
Comments, ideas, areas of improvement, questions, and constructive criticisms are welcome.
Feel free to contact me. Please be positive in your message.
I wish you the best.
Thank you.
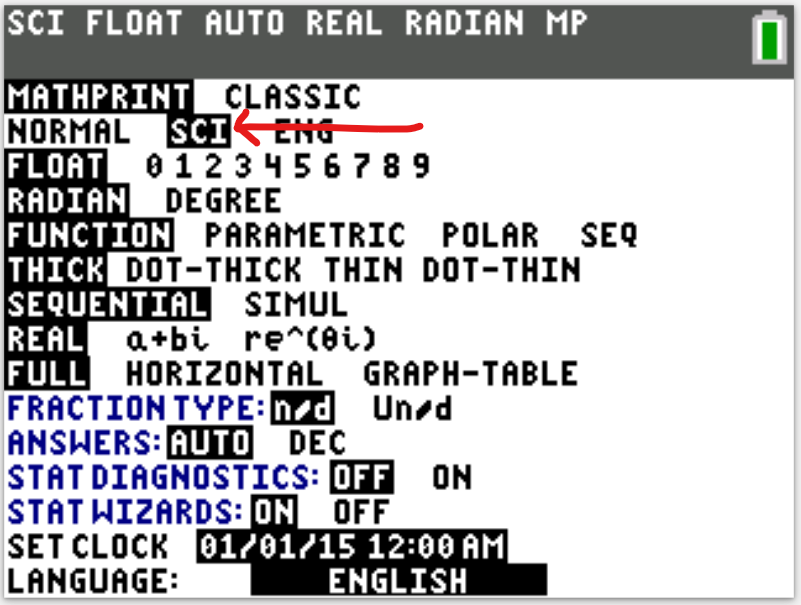
For calculations involving scientific notation as applicable, change the mode to SCI.
Percent Applications
$ \underline{\text{Change and Percent of Change}} \\[3ex] (1.)\:\: change = new - initial \\[5ex] (2.)\:\: \%\;\;of\;\;change = \dfrac{change}{initial} * 100 \\[5ex] (3.)\;\; \text{If A is p% more than B, then A = (100 + p)% of B} \\[3ex] (4.)\;\; \text{If A is p% less than B, then A = (100 – p)% of B} \\[5ex] \underline{\text{Percent:Proportion}} \\[3ex] (5.)\;\; \dfrac{is}{of} = \dfrac{\%}{100} \\[5ex] (6.)\;\;\underline{\text{Percent:Equation}} \\[3ex] is \rightarrow equal\;\;to \\[3ex] of\;\; \rightarrow multiply \\[3ex] what \;\; \rightarrow variable \\[5ex] \underline{\text{Wholesale and Retail}} \\[3ex] (7.)\:\: \text{Sale Price } = \text{Initial Price } - \text{Discount} \\[5ex] (8.)\:\: \%\;Discount = \dfrac{Discount}{\text{Initial Price}} * 100 \\[5ex] (9.)\;\; \text{Profit } = \text{Selling Price } - \text{Cost Price} \\[3ex] (10.)\;\; \%\;Profit = \dfrac{Profit}{\text{Cost Price}} * 100 \\[5ex] (11.)\;\; \text{Loss } = \text{Cost Price } - \text{Selling Price} \\[3ex] (12.)\;\; \%\;Loss = \dfrac{Loss}{\text{Cost Price}} * 100 $
Formula Sheet: List of Formulae
(i.) $\dfrac{2}{3} \;\;of\;\; \left(\dfrac{1}{8} + \dfrac{5}{12} \div \dfrac{1}{9}\right)$, giving your answer in EXACT form.
(ii.) $314.2 - \dfrac{26082}{52164}$, giving your answer in standard form.
(b.) Jim packed several cases of fruit juice for sale.
Each case contained 24 boxes of juice in 3 different varieties: apple, orange, and pineapple in the ratio 2:5:1 respectively.
(i.) How many boxes of pineapple juice were packed in each case?
(ii.) The profit gained from selling ALL of the boxes of pineapple juice is $35.64.
Each box of pineapple juice was sold at $3.34.
(a.) Show that the cost price of a box of pineapple juice is $2.35
(b.) Calculate the percentage profit made on the sale of the boxes of pineapple juice.
$ (a.) (i.) \\[3ex] \dfrac{2}{3} \;\;of\;\; \left(\dfrac{1}{8} + \dfrac{5}{12} \div \dfrac{1}{9}\right) \\[5ex] \underline{PEMDAS} \\[3ex] \text{Parenthesis: Division and Addition} \\[3ex] Division: \\[3ex] \dfrac{5}{12} \div \dfrac{1}{9} \\[5ex] = \dfrac{5}{12} * \dfrac{9}{1} \\[5ex] = \dfrac{15}{4} \\[5ex] Addition: \\[3ex] \dfrac{1}{8} + \dfrac{15}{4} \\[5ex] = \dfrac{1}{8} + \dfrac{30}{8} \\[5ex] = \dfrac{31}{8} \\[5ex] of \implies Multiplication: \\[3ex] \dfrac{2}{3} \;\;of\;\; \dfrac{31}{8} \\[5ex] = \dfrac{2}{3} * \dfrac{31}{8} \\[5ex] = \dfrac{31}{12} \\[5ex] (ii.) \\[3ex] 314.2 - \dfrac{26082}{52164} \\[5ex] = 314.2 - 0.5 \\[3ex] = 313.7 \\[3ex] = 3.137 * 10^{2}...\text{in standard form} \\[5ex] (b.)(i.) \\[3ex] \text{24 boxes containing 3 fruit varieties} \\[3ex] \text{Apple : Orange : Pineapple = 2 : 5 : 1} \\[3ex] \text{sum of ratios} = 2 + 5 + 1 = 8 \\[3ex] \text{number of boxes of pineapple juice} = \dfrac{1}{8} * 24 \\[5ex] = 3\;boxes \\[3ex] $ (ii.) (a.)
Selling Price, of each pineapple juice box = $3.34
Profit gained from selling all pineapple juice boxes = $35.64
But we are not given the number of pineapple juice boxes that were sold
To show that the cost price of a pineapple juice box = $2.35, we shall assume that 36 boxes of pineapple juice boxes were sold.
$ \underline{\text{36 boxes of pineapple juice}} \\[3ex] \text{Selling Price} = 3.34(36) = \$120.24 \\[3ex] Profit = \$35.64 \\[3ex] Profit = \text{Selling Price} - \text{Cost Price} \\[3ex] \text{Cost Price} = \text{Selling Price} - Profit \\[3ex] = 120.24 - 35.64 \\[3ex] = \$84.60 \\[3ex] \underline{\text{1 box of pineapple juice}} \\[3ex] C.P = \dfrac{84.60}{36} = \$2.35 \\[5ex] (b.) \\[3ex] \text{%Profit} = \dfrac{\text{Profit}}{\text{Cost Price}} * 100 \\[5ex] = \dfrac{35.64}{84.6} * 100 \\[5ex] = 42.12765957\% \\[3ex] \approx 42.1\%...\text{to 3 significant figures} $
(1.)(a.)(i.)
(ii.)
